Over the years, I've noticed certain difficulties that seem to be universal among students. There are certain concepts that are particularly difficult for them to grasp, many of which are basic ideas of numeracy and number manipulation. This lack of understanding has a tendency to fester over years and make students think that math is some sort of magical subject that works just because that's how it works. Two prime examples of this are manipulation of positive and negative numbers and almost anything dealing with fractions.
This is a huge problem for students and for society in general and one that I believe can be solved by better teaching. In many cases, these topics are seen as painful and hard and teachers gloss over them. We give students formulas or mnemonic devices to remember what to do (flip-change-change or two negatives make a positive) that get them through the test but don't offer deep numerical understanding and lead to problems later on when we cover more complex manipulations.
Since it's the beginning of Math 7 and Pre-Algebra, we are currently examining integer operations. Conversations with other educators, as well as my own experiences have moved me towards the idea of using counters and manipulative tools to illustrate the concepts of addition and subtraction of positive and negative integers.
Thankfully, I happen to have a giant bin of multicolored interlocking hexagonal blocks that I can have my students utilize for the benefit of their kinesthetic mathematical understanding.
So counters:
"I'm taking us on a field trip, to an actual field. In this field, I want to make piles of dirt. If I make a pile, and I didn't bring any dirt with me, what else am I going to have to make?"
"Holes?"
"Exactly! For every pile I make, I have to dig a hole. At the end of the trip, I push the piles back into the holes. What happens?"
"You're back where you started."
An extended version of this is how we introduce the concept of Zero Pairs, where 1 positive and 1 negative pair together to give us zero.
Before getting into anything complex, we start with addition, to get an understanding of the counters.
We write the expression at the top.
This problem starts with positive 4, so we take 4 yellow counters and put them on our card.
Since this expression is addition, we know that we need to add more counters to our card. The 3 tells us that we need to add 3 yellow counters.
We now look at the card and count the number of yellow counters, which in this case, is 7.
We did a few examples of this simple problem to get the kids used to moving the counters. Then we began working with the negative counters.
Again, we write the problem at the top.
Just as before, this problem tells us that we start with 5 positive counters, so we put them on our card.
Again, the addition sign says that we are going to add counters to the card, but this time, we will be adding 2 red counters, because each red counter represents negative 1.
Next, we line up our counters, creating pairs of opposite colors.
Just like with the piles of dirt and the holes in the ground, we can recognize that each pair of red and yellow counters is worth zero. The positive and negative counters cancel each other out.
With the zero pairs sectioned off, we examine what we have left, which is 3 yellow counters.
What I LOVED about doing this in class was how the students played with the hexagons to use them as they understood. There were so many ways that they made the zero pairs and the hexagons allowed them the freedom to explore those options.
"First I made them into rows. Then I stacked them on top of each other. Anywhere that had the hexagons together, stacked on top, I took them off the row. What I had left was my answer."
"I also made rows, but I put them together side by side. I pulled off the ones that had pairs."
"We started with stacks, so I left them as those stacks. When I put the stacks together, the pairs stayed together while the ones without pairs just spun off the top."
This method was, by far, my favorite!
To give them a chance to see if their methods worked, we did another problem.
Those were just addition problems. Do these methods work with subtraction as well? We tried one!
We wrote the problem at the top of the card.
Since the problem began with 5 yellow counters, we put them on the card.
Just as the addition symbol told us that we would be putting more counters on the card, the subtraction symbol tells us that we would be taking some away. The "-2" tells us that we need to take 2 positive counters away.
When we take those 2 counters away, we are left with 3 yellow counters, giving us an answer of positive 3.
"But Mr. Aion, what if you don't have enough counters to take away?"
I'm so glad you asked! Let's try an example of that!
Again, we start by putting 2 yellow counters on our card.
Uh oh! We're supposed to take 5 yellow counters away, but we don't have that many! What will we do!
Oh! I have an idea!
Right now, we have 2 on our card.

We already saw that if we put a red and yellow counter together, they have a value of zero.
That means I can add a pair of red and yellow counters to my card and STILL have a value of 2!
If 1 pair of red and yellow counters is worth zero, then 3 pairs would also be worth zero!
That means I can add 3 pairs of counters to my card and still have the value be 2!
Once those pairs are on my card, I can separate them into their positive and negative counters.
Our problem told us that we needed to take 5 positive counters off of the card. Since we started with only 2 and put on 3 pairs, we now have 5 yellow counters that we can remove.
After we remove the 5 positive counters, we are left with 3 negative counters, giving us a value of -3.
I really enjoyed working with the kids on this lesson and they loved playing with the hexagons. I was incredibly impressed with how they picked up the concepts and how they developed new ways of demonstrating what was going on.












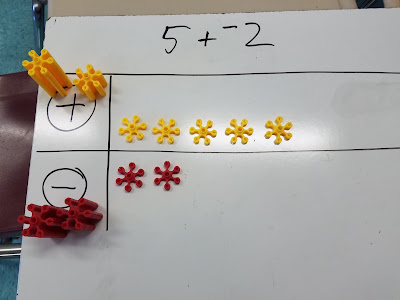
































Please! try my "Touch Integers" app maybe will be useful to you: https://play.google.com/store/apps/details?id=com.nummolt.touch.integers
ReplyDeleteIt can explain addition ,subtraction and multiplication.
For only addition and subtraction, there's the "Touch decimals" I am thinking and trying to explain this with computers and any kind of device from 20 years ago in my site nummolt.com Sometimes is useful to play with the background for to explain signs: A subtraction can be to borrow tokens, or add negative tokens. A negative token in a negative background, is positive...... (thanks)
I love this! I have a bunch of those hexi-blocks as well. This post almost makes me wish I was teaching middle school instead of 11th graders. Almost! :-)
ReplyDeletePS. I have been a really unreliable blogger, even though I have great intentions. I took a page from your book and I'm trying to blog every school day. You are my inspiration this year!
http://thiscatdoesmath.blogspot.com
You totally can! I've found that even the 11th and 12th graders love playing with them. You can have them examine patterns in 2 and 3 dimensions! What content are you teaching?
DeleteAnd your blog looks great!!